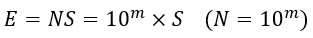
A new equation for calculating the energy of electromagnetic waves

The kinetic energy of objects is calculated by the following universally valid relation:

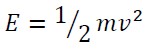
And it states that the amount of mass (m) multiplied by the square of the velocity (v) of any object always gives us its kinetic energy (E). It can also be used in electromagnetic waves so that in this relation, the mass is the same as the mass of the photon and the velocity is the same as the velocity of photon. Also, since the mass and velocity of photon are constant values, therefore the relative product of one-half of the mass (m), multiplied by the square of the photon velocity (C), is always a constant value. So the basic energy of electromagnetic waves can be defined using the following equation:

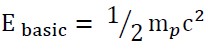
Since this term always is a constant value, we represent it by, “S”. In fact, “S” is the "basic unit of energy of electromagnetic waves". And its value is equal to:

Considering that “S” is always a constant value, to calculate the energy of various electromagnetic waves, it is enough to multiply the coefficient of that specific wave “n” by “S”.

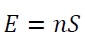
Where for each wave “n” or the same "energy coefficient of electromagnetic waves" is different. To obtain this coefficient for each electromagnetic wave, we do as follows:

For example, we calculate the energy coefficient of electromagnetic wave of green light (𝑛_𝐺) with a frequency of 555 THz:

In the same way “n” can be calculated for all electromagnetic waves.

Notice:

In general, it can be said that the energy of all particles in the universe, from the smallest to the largest ones, even the whole universe, consists of: