
New Calculation of Frequency and Energy of Magnetic Fields With Scientific Experiment, Nature, and Its Structural Model
1. Calculation of Frequency and Energy of the Magnetic Fields
2. Nature and Structure of Magnetic Fluxes
1. Calculation of Frequency and Energy of the Magnetic Fields
To calculate the frequency of the magnetic field, we use the following experiment: two annular magnets with identical characteristics are placed facing each other by the same poles. The upper magnet is in equilibrium thanks to the interactions between the force of its weight and the magnetic force of the lower magnet. Therefore, the gravitational potential energy of the upper magnet must be equal to the magnetic energy of the lower magnet. Then we measure the distance between the two magnets [1-3].
By multiplying this distance with the mass and the gravitational constant of the Earth (g=10) we obtain the gravitational potential energy of the upper magnet which is equal to the magnetic energy of the lower magnet. On the other hand, we consider the magnetic energy of the magnet as “nhϑ” and deal with the calculation of the frequency of the magnetic field of the magnet. So we have:
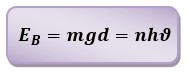
The above tests are repeated by different magnets [1-3].
Case 1: Both the lower and upper magnets are 2.5 grams:
The distance between the two magnets is 2.30 cm. Therefore, we have:

Case 2: Both the lower and upper magnets are 5 grams:
The distance between the two magnets is 2.32 cm. Therefore, we have:
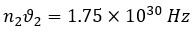
Case 3: Both the lower and upper magnets are 7.5 grams:
The distance between the two magnets is 2.33 cm. Therefore, we have:
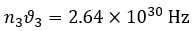
We continue the same approach and we have obtained the result presented in Table 1
Based on the above experiment, it can be concluded that:
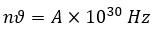
In which 1030 is our constant and there is a variable coefficient “A”

Table 1
Note 1:
As we know, force lines or magnetic fluxes are invisible, and on the other hand, they pass through objects. Considering these characteristics, we can certainly say that the frequencies of magnetic fluxes are obviously higher than those of visible waves. It can therefore be deduced that the start of the frequency range of the magnetic field must be 1015 Hz. So, in this test, the value of "n" is also around 1015.
We repeated the experiment with 45 grams of magnets, and the following results were obtained (Table 2):
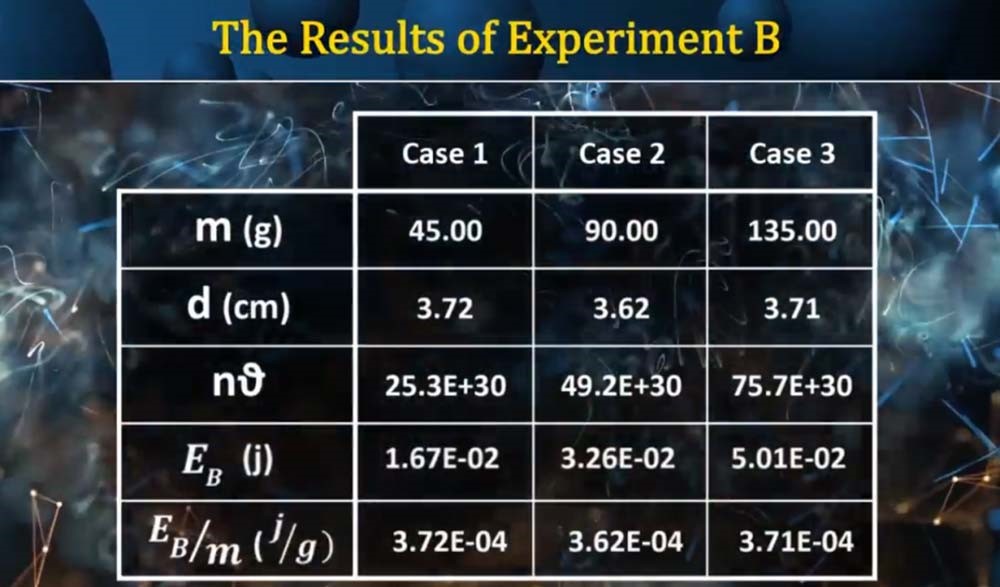
Table 2
In this experiment, we achieved similar results. So, it can be logically inferred that the beginning of the frequency range of magnetic waves should be 1015 Hz, and therefore the beginning of the range of "n" is also 1015. On the other hand, as you can see, the value of distance “d” is between 2.30 and 3.72 cm.
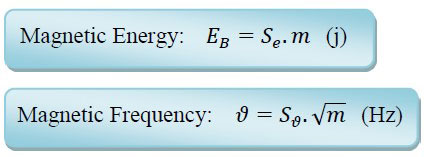
According to the formula obtained for the magnetic energy of the magnet (𝐸𝐵 = 𝑚𝑔𝑑) and by averaging the energies obtained in the experiments per gram of magnet, the energy of one gram of ordinary laboratory magnet can be considered approximately equal to 3×10-4 𝑗/𝑔. We call that the “Saleh Energy Constant” (𝑺e).

So, the amount of magnetic energy of an ordinary laboratory magnet is generally equal to:

Where “m” is mass in units of grams. Therefore, by measuring the mass of a magnet and using this formula, the energy of the magnet can be easily obtained.
Note 2:
I. Considering that 𝑛𝜗 always has a constant value of 1030, by increasing the mass of the magnet, the coefficient of 𝑛𝜗 will change, but its value will always remain constant at 1030.
II. Although by increasing the mass of the magnet, the number of magnetic fluxes “n” and magnetic field frequency will increase, this increase is such that the frequency remains in the range of 1015 to 1016 Hz.
Now we will study the dependency of magnetic frequency ϑ and the number of magnetic fluxes “n” to the mass. As was mentioned before, the frequency will remain in the range of 1015 to 1016 Hz. So, the number of magnetic fluxes can be defined as follows:
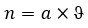
Where “a” is between "1" and "10"; therefore:
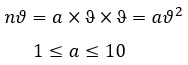
On the other hand, we obtained:
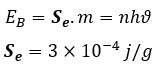
As a result:
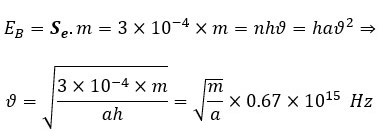
As we said before:
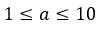
So:
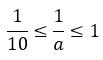
By averaging ⚊1a and putting it in the relation, we have:
The constant value is called the “Saleh Frequency Constant” (𝑺𝝑) and we have:
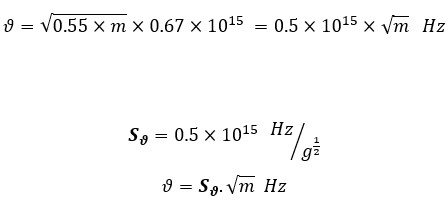
Where “m” is the mass of the magnet in units of grams. Therefore, by measuring the mass of the magnet and using this formula, the magnetic frequency of the magnet can be easily obtained. As a result, the energy and frequency of the magnets can be easily calculated using these two formulae [1-3]:
Note 3:
I. It should be noted that, as previously mentioned, by increasing the magnetic mass, in addition to the frequency, the number of magnetic fluxes will increase accordingly. However, considering the limitations of the frequency range of the magnetic field, it can be concluded that although the magnetic frequency of magnets is dependent on mass, it has limitations and will always remain in an approximate range of 1015 to 1016 Hz (Fig. 1). But the magnetic field energy of magnets, considering the wide range of variations for "n," may have lower limitations.
II. The accuracy of these formulae is about 97%.

2. Nature and Structure of Magnetic Fluxes
According to the relative penetrability of magnetic fields and their special and beautiful state, it can be said that magnetic waves are not single photons. Rather, they are a group of photons that are joined together in a chained state. For better conception, it can be said whenever we bring two like poles of the magnet closer to each other, the repulsion effect is seen, as if we have placed two invisible springs between them, and we are squeezing the springs. These springs are the same as continuous magnetic fluxes [4,5].
Due to this special form, it can be said that the structure of the magnetic field is similar to the structure of gravity flux and chained photons. Since in this model, toward the linear direction, the fluxes are firm and steady, and in the perpendicular direction, they have curvature property. These magnetic fluxes are made of photons, but the placement and structure of these photons are interconnected like chains. In fact, magnetic fluxes bend and compact and return to their original shape. So, it can be said that the magnetic field fluxes, similar to gravity fluxes, are made of interconnected photons.
References:
[3] Saleh, Gh. "New Discoveries about the Magnetic Fields." APS Meeting Abstracts. 2023.






