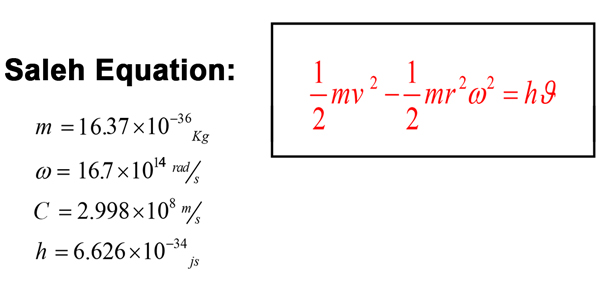
New Definition of Color Variety of Photons

New definition of color Variety of photons
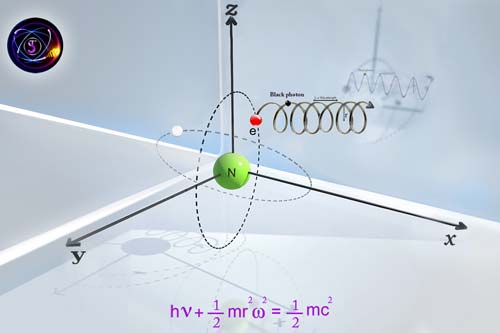
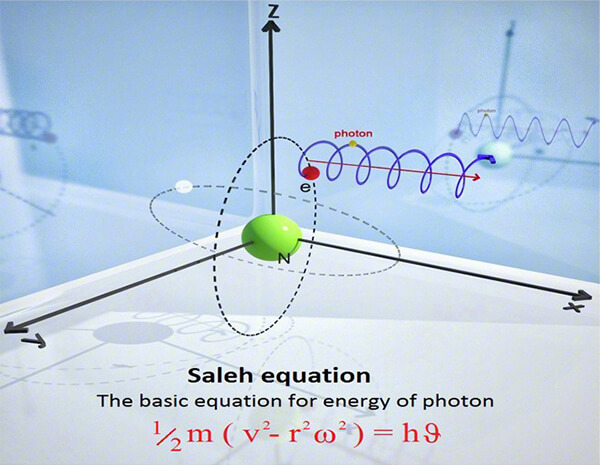
In Saleh Theory, we believe on the helical photon motion, of which r is the gyroradius around an imaginary axis. This means that the photon has two simultaneous motions: rectilinear motion at constant speed (C) and rotary motion at constant angular velocity (ω). (Fig. 1)

Fig.1
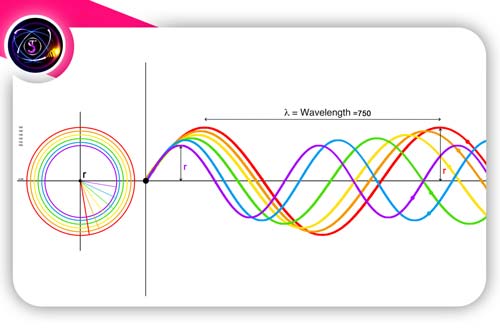
The visible spectrum is one of the concepts that have attracted much comment. In Saleh Theory the distinction of spectrums is due to the gyroradius; and its changes induce the colorfulness ( light color ). In fact, the increase of the gyroradius means reciprocally the widening of the wavelength. For example, the long wavelength (λ) of the red spectrum is a result of the high value of its gyroradius r, while for the blue spectrum, it’s the opposite. (Fig. 2)
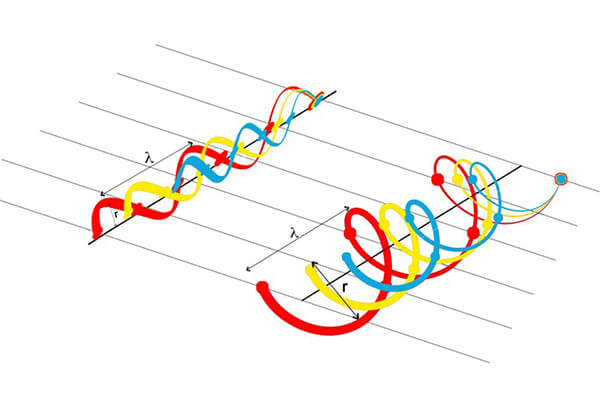
Fig.2
Saleh Theory, therefore, considers photon as a particle which generates different spectrum following variations in the gyroradius, and, indeed, the light color is the effect of the order of magnitude of r. (Fig. 3)

Fig.3
We know from the Earth that its angle of rotation around the sun conceptualizes the seasons. Similarly, the rotation of the photon around its imaginary axis projects the different light spectrum.
In fact, the emitted photon has an initial energy from which must be subtracted the Rotational kinetic energy to have the sum of the Translational kinetic energy ( light energy ):
Translational kinetic energy = Initial energy - Rotational kinetic energy
Saleh Theory knows the Translational kinetic energy, light energy, as the same energy that Thomas Planck had presented with his famous formula: E=hϑ measured empirically in the laboratory.
E = ⚊12 mv 2 - ⚊12 mW 2
W = rω
Saleh equation:
The basic equation for energy of photon
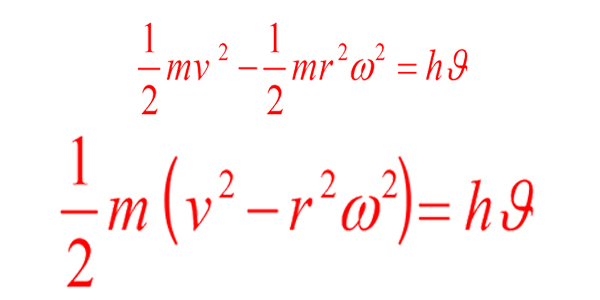
Where, m is the photon mass, v its straight velocity, r its gyroradius, h Planck constant, ϑ its frequency and ω its angular velocity. The angular velocity is the rate of velocity at which a photon is rotating around the center.
In first part of this equation for energy of photon v is the velocity of photon throw imaginary axis and equals speed of light C, and ω have the constant value in consumable part.
This explains that variety of light spectrum ( light color ) in Saleh Theory is the photon’s different gyroradius during its trajectory around an imaginary axis. (Fig. 4)
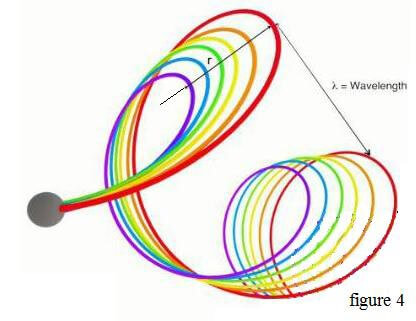
Fig.4
Attention:
r is directly related to amplitude and wavelength.
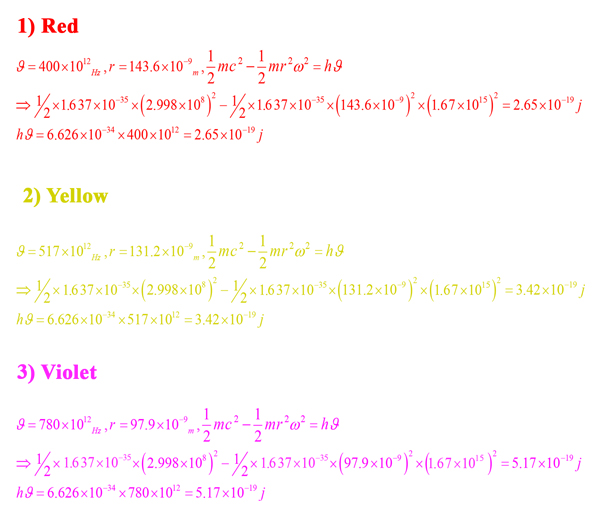
Therefore, assuming the mass of the photon m = 16.37 ✕ 10 -36kg and the approximate value of its angular velocity equal to ω = 16.7 ✕ 1014 rad/s, the gyroradius can be calculated for three spectrums; violet (λ=380nm), yellow (λ=580nm) and red (λ=750nm):
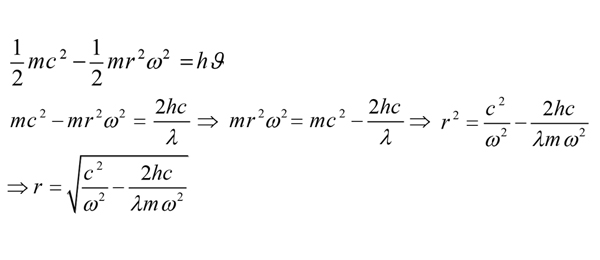
λ 1 = 380nm ⇒ ϑ = 780 ✕ 10 12 Hz ⇒ r = 97.9nm
λ 2 = 580nm ⇒ ϑ = 517 ✕ 10 12 Hz ⇒ r = 131.2nm
λ 3 = 750nm ⇒ ϑ = 400 ✕ 10 12 Hz ⇒ r = 143.6nm
Given the mass value of photon and ω, the gyroradius for the visible spectrum should be between 100nm and 150nm.
See figure 5 to have better 3D sense about helical photon motion.
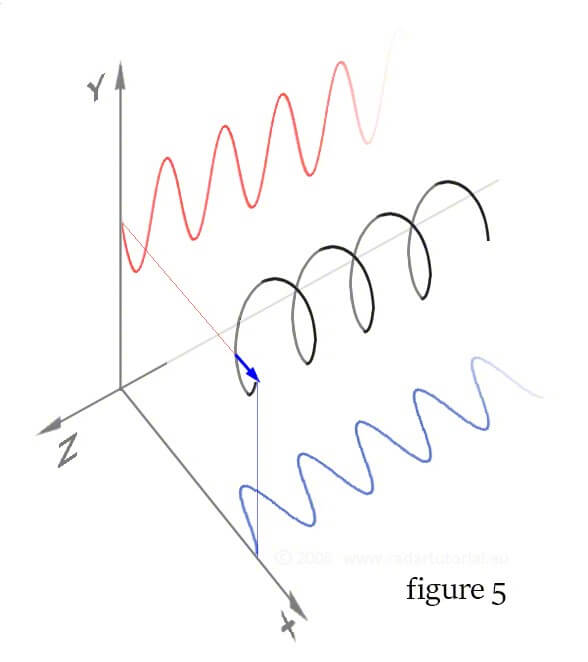
Fig.5